NBSE GUIDE ONLINE

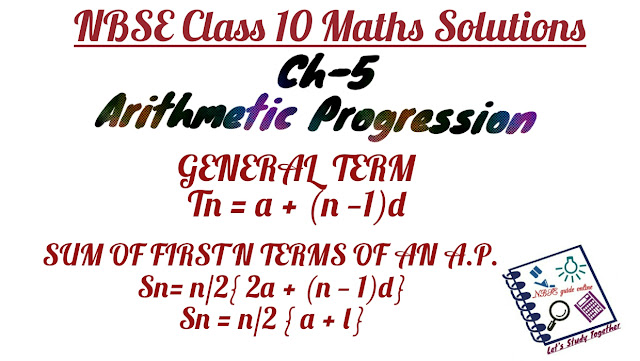
ARITHMETIC PROGRESSION  This chapter introduces students to a new topic that is  Arithmetic Progression i.e A.P. Here, students will learn to represent a situation or problems in the form of A.P, finding the first term and difference of an A.P, finding out whether a series is A.P or not. The chapter goes on to explain how to find out the nth term of an AP by using the following formula; a n  = a + (n-1) d and also how to find sum of first n terms of an A.P using the two different formula : Sn= n/2{ 2a + (n — 1)d} Sn = n/2 { a + l} The chapter ends with higher-level questions based on AP to enhance students analytical and power solving skills. To download Chapter 5 (Arithmetic Progression) solutions in PDF for future use please click on the link below : Ex - 5.1 Download PDF Ex -  5.2 Download PDF Ex -  5.3 Download PDF Ex -   5.4 (optional) Download PDF Note : All the copyright of this PDF content belongs to NBSEguideonline. It is available to all N

Linear Equations In Two Variables This chapter will introduce the students to the linear equation in two variables, i.e., ax + by + c = 0. Students will also learn to plot the graph of a linear equation in two variables. ★★★ To download Chapter 4 (Linear Equations In Two Variables) solutions in PDF for future use please click on the link below. Ex - 4.1 : Download PDF Ex - 4.2 : Download PDF Ex - 4.3 : Download PDF Ex - 4.4 : Download PDF Note : All the copyright of this PDF content belongs to NBSEguideonline. It is available to all NBSEguideonline users without any subscription. ★★★ Points To Remember Linear Equations In Two Variables An equation of the form ax + by + c = 0 where a, b, c are real numbers and x, y are variables, is  called a linear equation in two variables. Here ‘a’ is called coefficient of x, ‘b’ is called coefficients of y and c is called constant term. Eg. 6x + 2y + 5 = 0, 5x – 2y + 3 = 0 etc. Solution Of a Linear Equation Let ax + by + c = 0 be a given linear