Class-10 : Maths - Chapter : 5 Arithmetic Progression (Ex-5.1 Ex-5.2 Ex-5.3 Ex-5.4 optional)
ARITHMETIC PROGRESSION
This chapter introduces students to a new topic that is Arithmetic Progression i.e A.P. Here, students will learn to represent a situation or problems in the form of A.P, finding the first term and difference of an A.P, finding out whether a series is A.P or not. The chapter goes on to explain how to find out the nth term of an AP by using the following formula; an = a + (n-1) d and also how to find sum of first n terms of an A.P using the two different formula :
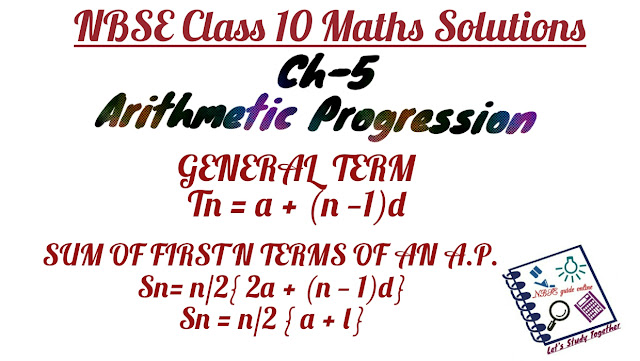
Sn= n/2{ 2a + (n — 1)d}
Sn = n/2 { a + l}
The chapter ends with higher-level questions based on AP to enhance students analytical and power solving skills.
Sn= n/2{ 2a + (n — 1)d}
Sn = n/2 { a + l}
The chapter ends with higher-level questions based on AP to enhance students analytical and power solving skills.
To download Chapter 5 (Arithmetic Progression) solutions in PDF for future use please click on the link below :
Ex - 5.1 Download PDF
Ex - 5.2 Download PDF
Ex - 5.3 Download PDF
Note : All the copyright of this PDF content belongs to NBSEguideonline. It is available to all NBSEguideonline users without any subscription.
★★★
POINTS TO REMEMBER
SEQUENCE
A collection of numbers arranged in a definite order according to some definite rule (rules) is
called a sequence. Each number of the sequence is called a term of the sequence. The sequence is called finite or infinite according as the number of terms in it is finite or infinite.
ARITHMETIC PROGRESSION
A sequence is called an arithmetic progression (abbreviated A.P.) if and only if the difference
of any term from its preceding term is constant.
A sequence in which the common difference between successors and predecessors will be constant. i.e. a, a+d, a+2d.
This constant is usually denoted by ‘d’ and is called common difference. The ‘d’ can be positive, negative or zero.
SOME EXAMPLES OF A.P
(a) The heights (in cm) of some students of a school standing in a queue in the
morning assembly are 147 , 148, 149, . . ., 157.
(b) The minimum temperatures (in degree celsius) recorded for a week in the
month of January in a city, arranged in ascending order are – 3.1, – 3.0, – 2.9, – 2.8, – 2.7, – 2.6, – 2.5
(c) The balance money (in ₹) after paying 5 % of the total loan of ₹ 1000 every
month is 950, 900, 850, 800, . . ., 50.
(d) The cash prizes (in ₹) given by a school to the toppers of Classes I to XII are,
respectively, 200, 250, 300, 350, . . ., 750.
(e) The total savings (in ₹) after every month for 10 months when 50 are saved
each month are 50, 100, 150, 200, 250, 300, 350, 400, 450, 500.
nth TERM OF AN A.P.
It is denoted by tn and is given by the formula, tn = a + (n —1)d where ‘a’ is first term of the series, n is the number of terms of the series and ‘d’ is the common difference of the series.
NOTE : An A.P which consists only finite number of terms is called a finite A.P. and which contains infinite number of terms is called infinite A.P.
REMARK : Each finite A.P has a last term and infinite A.Ps do not have a last term.
RESULT: In general, for an A.P a1 , a2, , an, we have d= ak + 1 — ak where ak + 1 and ak are the
(k+ 1)th and the kth terms respectively.
SUM OF FIRST N TERMS OF AN A.P.
It is represented by symbol Sn and is given by the formula,
Sn = n/2{ 2a + (n — 1)d} or,
Sn = n/2 { a + l} ; where ‘l’ denotes last term of the series and
l = a+(n-1)d
REMARK : The nth term of an A.P is the difference of the sum to first n terms and the sum to first (n — 1) terms of it.
ie, an = Sn — Sn – 1.
TO FIND nth TERM FROM END OF AN A.P. :
nth term from end is given by formula :
l – (n –1)d
nth term from end of an A.P.
= nth term of (l, l — d, l – 2d,…….)
=l+(n-1)(—d)
=l—(n-1)d
PROPERTY OF AN A.P. :
If ‘a’ , b, c are in A.P., then
b — a= c — b or 2b= a + c
THREE TERMS IN A.P. :
Three terms of an A. P. if their sum and product is given, then consider
a—d, a, a+d.
FOUR TERMS IN A.P. :
Consider a —3d, a — d, a+ d, a +3d.
NOTE :
The sum of first n positive integers is given by Sn= n(n + 1) / 2
★★★
NBSE Solutions for Class 10 Maths for all Chapters by NBSE Guide Online are provided here. Just click on the chapter wise links given below :
• Chapter 6 Triangles
• Chapter 7 Coordinate Geometry
• Chapter 8 Introduction to Trigonometry
• Chapter 9 Some Applications of Trigonometry
• Chapter 10 Circles
• Chapter 11 Constructions
• Chapter 12 Areas Related to Circles
• Chapter 13 Surface Areas and Volumes
• Chapter 14 Statistics
• Chapter 15 Probability
The solution list comprises all the chapter-wise answers to the questions present in the NBSE book for Class 10 in a very precise and lucid manner, maintaining the objective of textbooks.
★★★
Related Links
★★★










Comments
Post a Comment
If you have any doubts please let me know